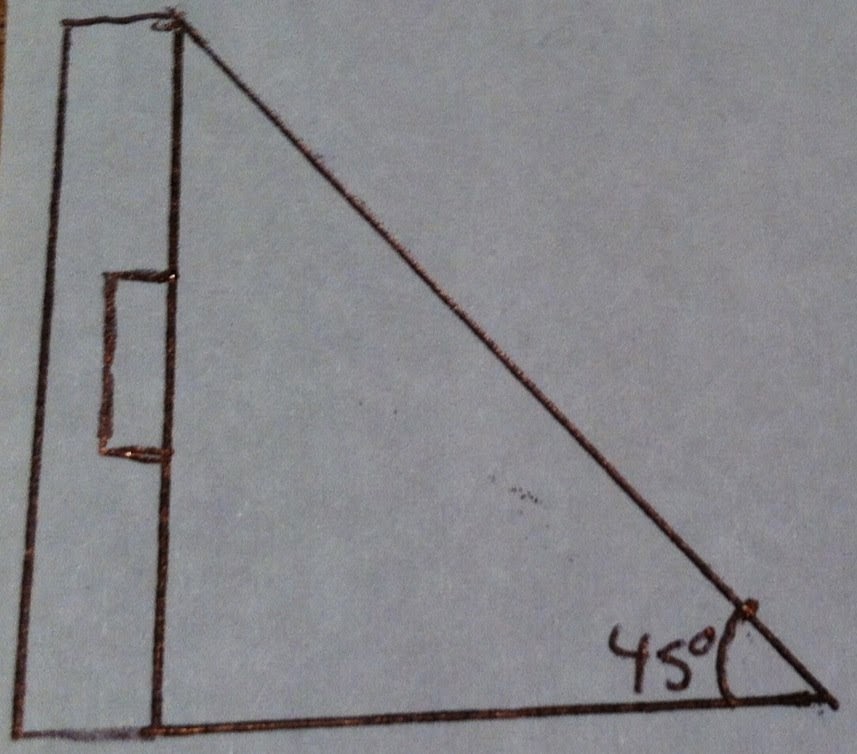
Sergio's Problem
Mark arrives at a rather tall building with the rest of his band (to sign a record deal). He is standing 50 feet away from the building, when he looks up, and sees a large He-man banner hanging from one of the windows, and thinks to himself: "Well, would you look at that." If the angle of elevation to the top of the building is N 45° W degrees, and the angle of elevation to the poster is N 25° W degrees, at what height is the banner hung?
Our first step in solving this, would be to figure out that the height of the wall is of no relevance - Sergio's fault - yet still, draw a picture of it.
Our next step would be to include the poster and label our given values (50 feet and 25°), and from there, calculate the other angle, since Sergio had decided to simplify our lives and make this a right triangle, which would leave the other angle as 65°.
Lastly, we would utilize the Law of Sines in order to match up 65° with 50, and make sin 65° / 50 equal to sin 25° / x, which, cross-multiplied and simplified, would lead to 23.3 feet as the height of the poster.
Brian's Problem
He-Man smiles upon his admirers, and although he was just a a guy on a poster, his bright smile reflects the sunlight, and shines the radiance upon Mark and the rest of his band. Somehow, he finds that the angle from which He-Man's smile shined light upon his buddies and him, was 115° (navigation method), and that the lengths that had sandwiched that angle, were 35 feet and 25 feet. What length of area did that light cover?
Our first step would be to draw our triangle and label all of the parts, in order to help us organize our next actions.
He-Man smiles upon his admirers, and although he was just a a guy on a poster, his bright smile reflects the sunlight, and shines the radiance upon Mark and the rest of his band. Somehow, he finds that the angle from which He-Man's smile shined light upon his buddies and him, was 115° (navigation method), and that the lengths that had sandwiched that angle, were 35 feet and 25 feet. What length of area did that light cover?
Our first step would be to draw our triangle and label all of the parts, in order to help us organize our next actions.
Our next and last step, would be to plug in all of our values into the Law of Cosines, simplify, and find our answer.